Simple and Compound Interest
- Math Lobby
- Jun 21, 2020
- 4 min read
Updated: Jun 22, 2021
In this note, you will learn:
1. The definition of simple interest and its calculations 2. The definition of compound interest and its calculations
Math Lobby believes that you have been to shopping malls or train stations and seen bank advertisements on the walls, many a times you will observe things like “Best Interest Savings Plan” or “High Interest Guaranteed!”, but what do they actually mean?
By definition, interest rate in a savings plan refers to the monetary returns based on an initial amount you placed into a bank, which is referred to as your principal amount. Interest rates are typically calculated in the form of percentage per annum, or in layman terms, per year.
This means that based on the principal amount you entrust the bank with, they have to pay you a specific amount every year, depending on the interest rate promised when you first registered for the savings plan.
There are two types of interests we will be learning today: Simple and Compound. By the end of this article, comment down below which method of interest you would prefer to benefit you if you were to open a bank account yourself!
Definition of simple interest and its calculations
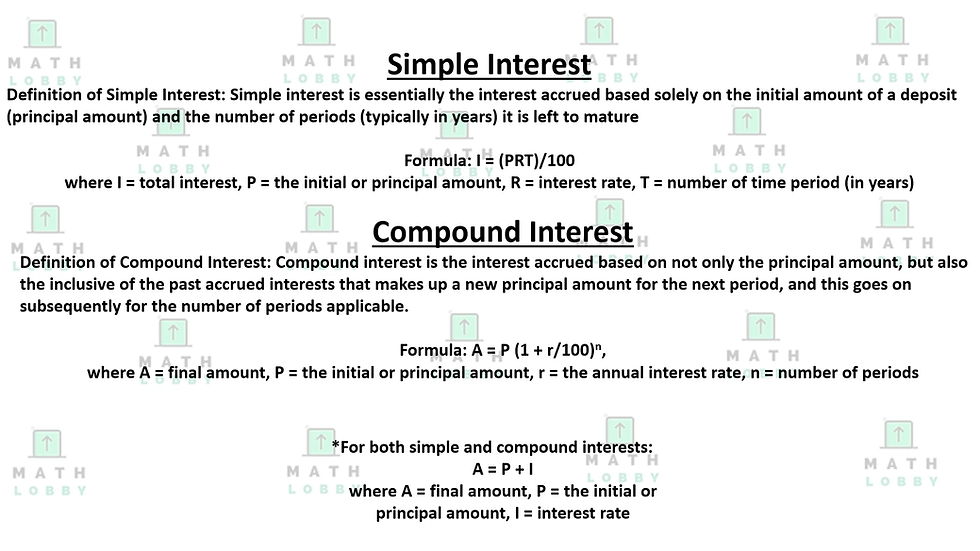
Simple interest is essentially the interest accrued based solely on the initial amount of a deposit (principal amount) and the number of periods (typically in years) it is left to mature. It is given by the formula:
I = (PRT)/100
where I = total interest, P = the initial or principal amount, R = interest rate, T = number of time period (in years)
*Another thing to note for both simple and compound interest is that the total amount after the calculation regardless if its simple or compound interest is given by the formula below:
A = P + I
where A = final amount, P = the initial or principal amount, I = interest rate
Let’s say that you have $5000, and you decided that you want to apply for a savings plan that offers you a simple interest rate of 5% per annum. What is the accrued interest after the 3 years? And how much will you receive in total after keeping that $5000 for 3 years in the plan?
First of all, let’s identify the respective terms in the question. It states that we have an initial amount of $5000, hence that would be our principal amount, P. Then, we are offered a simple interest rate of 5% per annum, hence it means that the annual interest rate, r, is 5%. Lastly, the number of periods is said to be 3 years, which means t = 3. Now, let’s try applying the formula:
I = (PRT)/100
I = [(5000)(5)(3)]/100
I = 750
Therefore, this means that we will receive an accrued interest of $750 by the end of the 3 years.
A = P + I
= 5000 + 750
= 5750
The total amount we will receive after the 3 years is $5750.
The definition of compound interest and its calculations
Compound interest, on the other hand, is the interest accrued based on not only the principal amount, but also the inclusive of the past accrued interests that makes up a new principal amount for the next period, and this goes on subsequently for the number of periods applicable. The formula is defined as:
A = P (1 + (r/100))^n,
where A = final amount, P = the initial or principal amount, r = the annual interest rate, n = number of periods
This might be slightly confusing right from the get go, but let’s try to understand this with an example:
Given that John takes a bank loan for his studies in the university, which is $40000. The bank charges a compound interest of 0.5% per annum that is compounded once every four months. Provided that John wants to finish paying his debts in three years’ time, how much will he need by the end of the three years?
The initial principal amount is $40000 with a compound interest rate of 0.5% per year, and the number of times interest is applied in the time period of one year, will be 3 since interest is compounded once every four months (12 months in three years / 4 months = 3 times), and in a span of three years, total number of periods would be 9 ( 3 times x 3 years = 9)
Now, let’s try applying the formula:
A = P (1 + (r/100))^n
A = (40000) [1 + ((0.5) / (100))]^(9)
A = 40000 (1.03)^9
= 41836.42 (2 d.p)
Hence, John will need to have $41836.42 by the end of the three years to clear his debt.
Question Time!
1. Given that Timmy has $2000 in his savings and decided to place it in a bank that offers an interest rate of 4% per annum. Calculate the total amount he will receive after five years, and the total accrued interest.
2. Jane decides to invest in a company during this economic recession, and deposits $5000 to buy their shares. The company is to offer its’ shareholders a dividend every 3 months and Jane decides to re-invest the dividends in the same company every time to compound her dividends. Given that the amount of dividends Jane receives in a year is 6% of her initial deposit, calculate how much Jane will receive after 3 years assuming that the prices of the shares remains constant, and how much profits will be accrued over this period of 3 years?
And that’s all we have for today, students! Math Lobby hopes that you have a clear understanding of simple and compound interest after what we went through today, and if you have any questions or inquiries, feel free to contact Math Lobby on our website, Facebook or Instagram page! As always: Work hard, stay motivated and we wish all students a successful and enjoyable journey with Math Lobby!
If you want to receive daily Secondary Math Tips from us,
Visit and LIKE our Facebook page at www.facebook.com/mathlobbymotivation/
Visit and FOLLOW our Instagram page at https://www.instagram.com/mathlobbymotivation/
Visit our Website at: www.mathlobby.com
Contact us via SMS/WhatsApp/Telegram/Call +65 96322202
*
*
*
#mathlobby #mathlobbymotivation #bestmathtuition #mathtuitionpotongpasir #mathtuitionsg #freemathresources #sgmaths #sgmathtutor #emath #amath #secschool #mathtutorsg #sgedu #nleveltuition #mathtuition #secondarymath #mathtutors #tuitionsg #singaporemath #sgtuition #sgstudents #sgmath #singaporetuition #mathstuition #math #maths #mathtutor #tuition #sgparents #secondaryschool
